Marginal revenue product is the additional revenue generated by the use or employment of an extra variable input. It is closely related to the concept of marginal product (or marginal physical product). Marginal physical product indicates how much total production changes by employing another unit of variable input. Marginal revenue product, however, indicates how much total revenue changes by employing another unit of variable input. Marginal revenue product is also closely related to the concept of marginal revenue. Marginal revenue is the change in total revenue that results from changing the quantity of output produced.Marginal revenue product is an essential component of factor market analysis and marginal productivity theory. The extra revenue generated by an input is THE key influence on the price an employer is willing and able to pay to hire the input. An input with a greater marginal revenue product is bound to receive a higher price, payment, or income than one with a lower marginal revenue product.
Key to the analysis of factor demand, marginal revenue product is guided by the law of diminishing marginal returns. As marginal product decreases so too does marginal revenue product. But as marginal revenue product declines, employers are willing to pay less. This gives rise to the primary implication of marginal revenue product--the factor demand curve is negatively sloped.
Marginal revenue product can be derived as the change in total revenue due to a change in the variable input, as specified by this equation:
| marginal revenue product | = | change in total revenue
change in variable input |
Three (or Four) Marginals
Marginal revenue product is one of three (or four) related marginal concepts.- Marginal Product: This is the change in total product resulting from an incremental change in the quantity of the variable factor input used.
- Marginal Physical Product: This is another term for marginal product which serves to emphasize that production is measured in physical units rather than monetary units.
- Marginal Revenue: This is the change in total revenue resulting from an incremental change in the quantity of the output produced.
- Marginal Revenue Product: This is the change in total revenue resulting from an incremental change in the quantity of the variable factor input used.
Marginal revenue product is marginal product stated in monetary units rather than physical units. Rather than stating productivity of an input in terms of the physical quantity of production, marginal revenue product states productivity in terms of the revenue generated.Suppose, for example, that Edgar Millbottom contributes 5 tacos per hour of production when hired by Waldo's TexMex Taco World. Edgar's marginal (physical) product is thus 5 tacos per hour. However, because each taco sells for $2 each (marginal revenue), Edgar contributes $10 per hour of revenue to Waldo's TexMex Taco World.
Waldo, the owner of Taco World, is more interested in the amount of revenue Edgar generates when it comes to making out a paycheck, than just the number of tacos produced.
This connection between marginal product, marginal revenue, and marginal revenue product is summarized in by the following equation:
marginal
revenue product | = | marginal
product | x | marginal
revenue |
Marginal physical product, marginal revenue, and marginal revenue product are related beyond this equation. This relation reflects the basic production process of a profit-maximizing firm. A firm uses an input to produce output and the output is then sold. The firm transforms inputs into outputs according to constraints imposed by a production function. The firm then generates revenue by selling this output to wants-and-needs-satisfying buyers.
This process can be separated into two different links.
- Marginal (physical) product focuses on the first link, the physical input-output production relationship--the change in output resulting from a change in the input.
- Marginal revenue focuses on the second link, the revenue generated from selling the output.
Marginal revenue product combines both links, showing the relationship between the input and the revenue generated from selling the output, output produced by the input.The Value of Taco Production
| Marginal Taco Product | 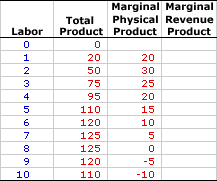 |
This table presents the hourly production of Shady Valley's favorite lunch time meal, Super Deluxe TexMex Gargantuan Tacos (with sour cream and jalapeno peppers), which can be used to investigate marginal revenue product. The column on the left is the variable input, specifically the number of workers employed by Waldo's TexMex Taco World, which ranges from 0 to 10. The column in the middle is the total product, the total number of TexMex Gargantuan Tacos produced each hour, which ranges from a low of 0 to a high of 125 before declining to 110. Note that the taco production from these workers depends on a given amount of fixed inputs--Waldo's TexMex Taco World restaurant and all of the capital that goes with it.The third column presents marginal physical product. The marginal physical product of the first worker is 20 tacos, the marginal physical product of the second worker is 30 tacos, for the third worker it is 25 tacos, etc.
The far right column, which is currently empty, is available to display marginal revenue product. The first step needed to derive the marginal revenue product of taco production is to identify the marginal revenue of taco sales. Because Waldo's Taco World is relatively competitive, it sells tacos at a constant price of $2. As such, 20 tacos generates $40 of revenue, 30 tacos generates $60 of revenue, etc.
With this information in hand, marginal revenue product can be derived by multiplying marginal physical product by marginal revenue (or the price of tacos). For an official display of all marginal revenue product values, click the [Marginal Revenue Product] button. The marginal revenue product of the first worker is $40, the marginal revenue product of the second worker is $60, for the third worker it is $50, etc.
The proper economic interpretation of marginal revenue product is the contribution of the last unit of variable input to total revenue. It answers the question: How much does total revenue change by adding the last unit of input? If, for example, total daily revenue received from selling tacos increases from $100 to $150 when an employee such as Edgar begins working at Taco World, then the conclusion is that Edgar's marginal revenue product is $50. Does this mean that Edgar personally produces the tacos that generate this extra $50? No, not at all. Or at least, not necessarily. It only means that having Edgar working causes total revenue to increase by $50. It could be that Edgar just makes other workers more productive.
Perhaps the most important observation is that marginal revenue product declines after reaching a peak of $60 for the second worker. This declining marginal revenue product corresponds with declining marginal physical product. And marginal physical product declines due to the law of diminishing marginal returns.
Marginal Revenue Product Curve
| Marginal Revenue Product Curve | 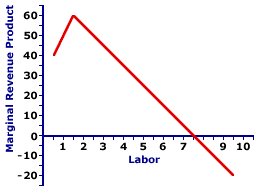 |
The marginal revenue product concept is one step on the path to understanding factor demand. The next step is the marginal revenue product curve.The marginal revenue product curve graphically illustrates the relation between marginal revenue product and the quantity of the variable input, holding all other inputs fixed. The curve displayed to the right is the marginal revenue product curve for taco production at Waldo's TexMex Taco World. It indicates the incremental change in total revenue for incremental changes in the variable input.
The shape of this curve is perhaps the most important thing to note. For the first two workers of variable input, marginal revenue product increases, as each added worker contributes more to total revenue than previous workers. This is reflected in a positive slope of the marginal revenue product curve. From the third worker on, the marginal revenue product declines, as each added worker contributes less to total revenue than previous workers. This is seen as a negative slope.
The hump-shape of the marginal revenue product curve embodies the essence of the analysis of short-run production. The upward-sloping portion of the marginal revenue product curve, up to the second worker, is due to increasing marginal returns. Decreasing marginal returns sets in after the marginal revenue product curve peaks with the second worker and declines for the third worker. In particular, this declining segment of the marginal revenue product curve reflects the law of diminishing marginal returns.
MARGINAL REVENUE PRODUCT, AmosWEB Encyclonomic WEB*pedia, http://www.AmosWEB.com, AmosWEB LLC, 2000-2024. [Accessed: October 30, 2024].


